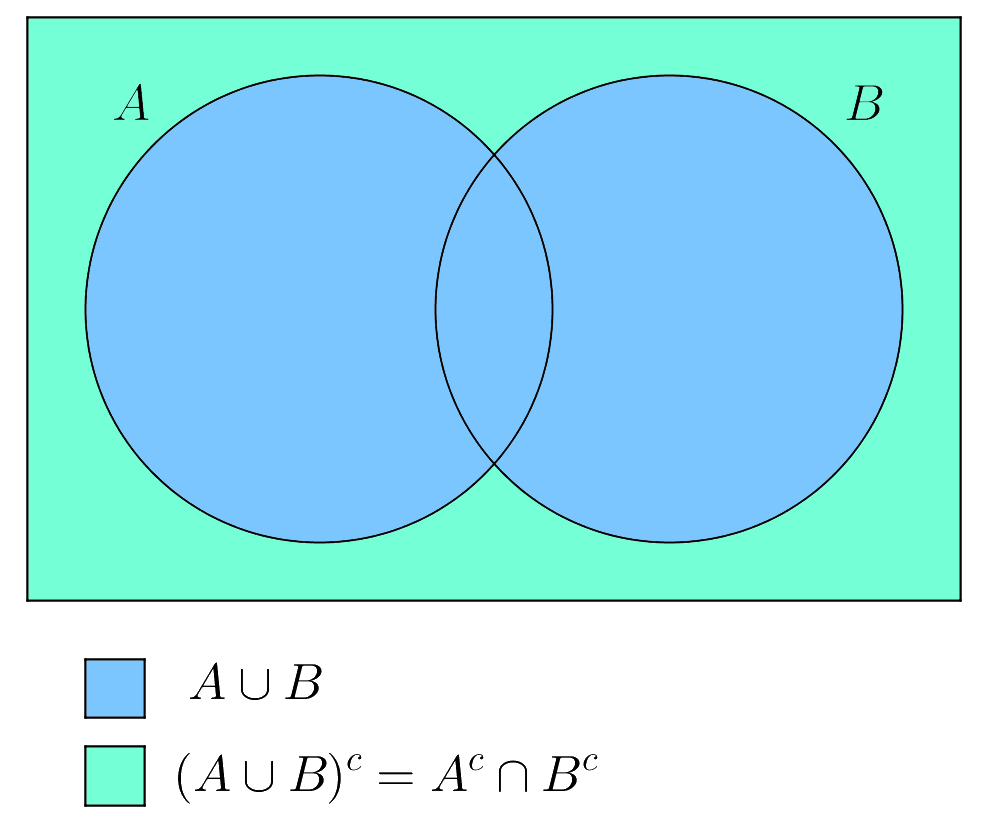
Prove De Morgan S Law Venn Diagram

De morgan s laws in set theory states that complement of the union of two sets is equal to the intersection of co. Answer to using venn diagrams prove de morgan s law a b a b. Complement of an intersection of two sets.

discrete mathematics venn diagram examples finding probability using venn diagrams differences between elements and compounds venn diagram ecological succession venn diagram answers euler diagram python draw venn diagram online free example problem of universal set finding hcf and lcm using venn diagrams tes

De Morgans Law Proof With Examples Set Theory Teachoo

Demorgans Law

Use Venn Diagrams To Verify De Morgan S Law Of Complementation A B A B

Discrete Mathematics De Morgan Law Proving By Venn Diagram 15 Youtube

Sets Venn Diagram Demorgan S Law Youtube

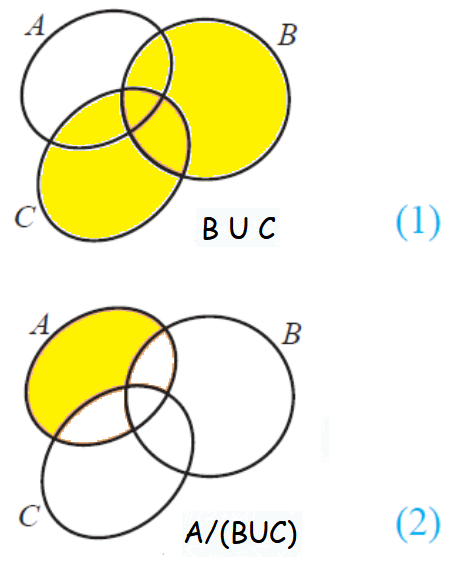
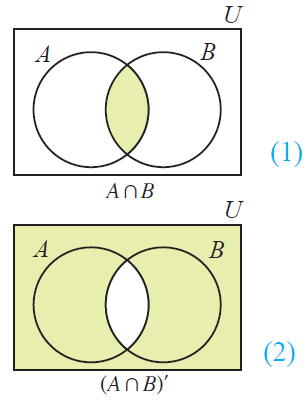
Proofs For De Morgan S Laws

A b n c a b u a c hence de morgan s law for set difference is verified.

Prove de morgan s law venn diagram. Using venn diagrams to verify set identities including de morgan s law the probability identity of either of two events happens or both happen by definition the sample space contains all possible outcomes of an experiment. Observe the union of the complements of two sets. So first we will make venn diagram of which is complement of so the venn diagram is. Let us take the first part of this equation and represent it in a venn diagram.

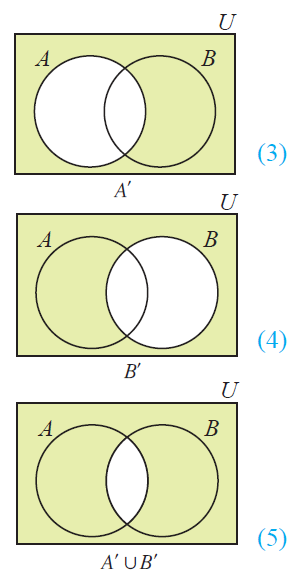
Proof by venn diagram. De morgan s law for set difference. This article explains the de morgan laws with the help of venn diagrams. A n b a u b from the above venn diagrams 2 and 5.

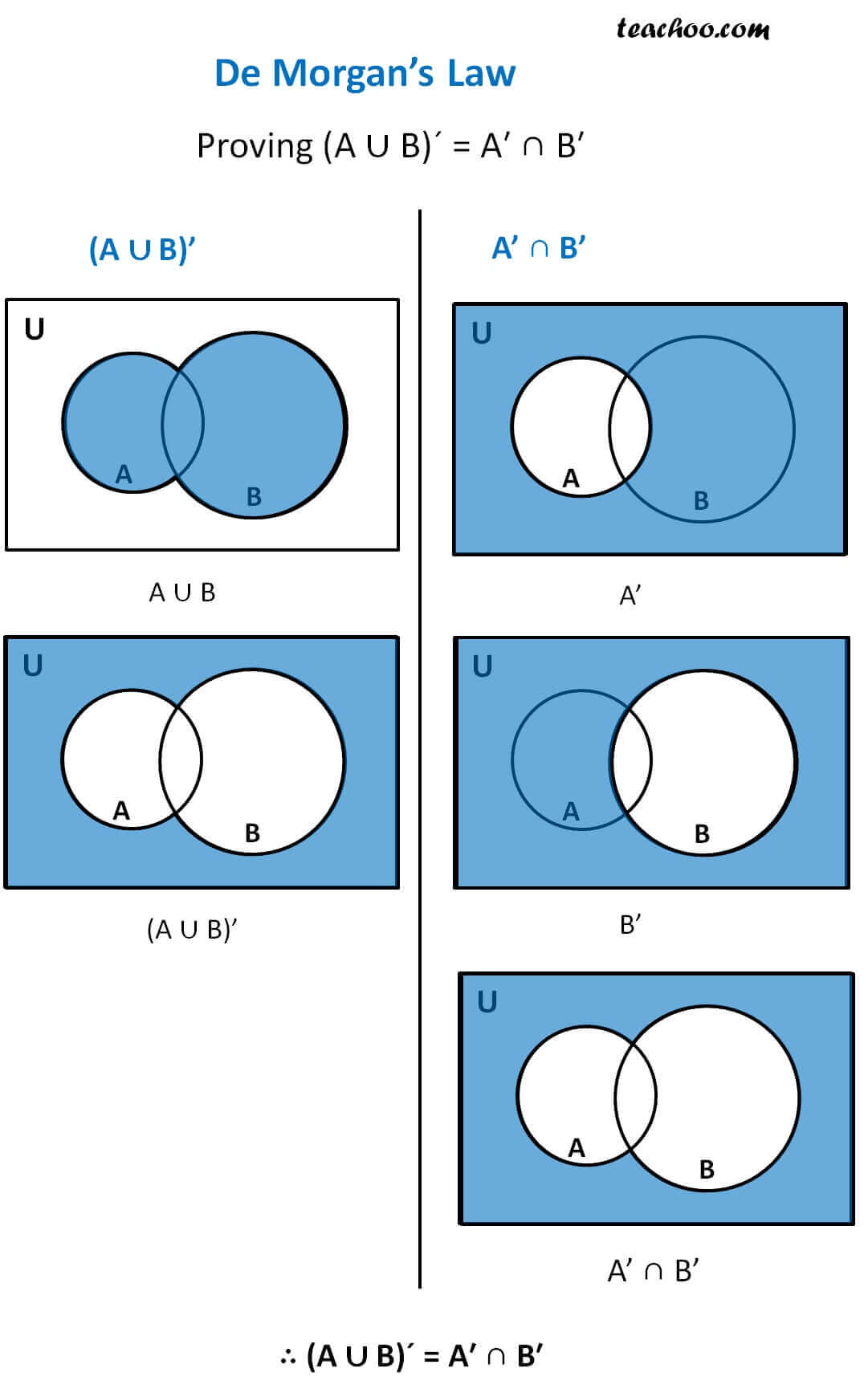
Learn the explanation to de morgan s laws. After having gone through the stuff given above we hope that the students would have understood proof by venn diagram. Augustus de morgan 1806 1871 was born in madurai tamilnadu india. The de morgan s law states that so it means the venn diagram of will be same as the venn diagram of then de morgan s law is proved.

Then i a u. For any three sets a b and c we have i a b u c a b n a c ii a b n c a b u a c de morgan s law for set complementation. A b n c a b u a c from the above venn diagrams 2 and 5 it is clear that. De morgan s law are based oncomplement of sets a b a b a b a b let us prove the law by venn diagramslet s take two sets a and b likeproving a b a b proving a b a b.

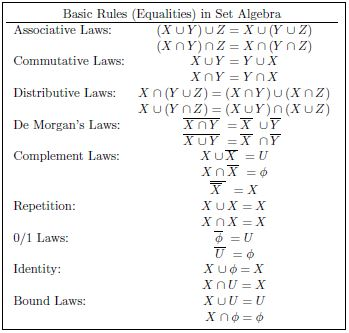
An easy way to visualize these rules is through venn diagrams. Consider set a and set b. De morgan s father a british national was in the service of east india company india. On a venn diagram this union covers all space in the venn diagram except for the intersection of the two sets.

Let u be the universal set containing sets a and b. He had his education at trinity college cambridge england. His family moved to england when he was seven months old. Applied to set theory de morgan s law states let s dig deeper into this law.

Hence de morgan s law for the complement of an intersection of two sets. From the above venn diagrams 2 and 5 it is clear that a n b a u b hence de morgan s law for complementation is verified. Now let us look at the venn diagram proof of de morgan s law for complementation. Now to the second part of the law which is the same as.

Here we are going to see the proof of de morgan s laws by venn diagram.